The Role of Argumentation Theory in the Logics of Judgements

Fig. 32.1
The Semantic Web stack of technologies
a document metadata structure, capturing the main parts of the judgement to create a bridge between text and semantic annotation of legal concepts;
a legal core ontology, describing the legal domain’s main elements in terms of general concepts through an LKIF-Core extension;
a legal domain ontology, an extension of the legal core ontology representing the legal concepts of a specific legal domain concerned by the case-law, including a set of sample precedents;
argumentation modelling and reasoning, representing the structure and dynamics of argumentation.
The research is based on a middle-out methodology: top-down for modeling the core ontology, bottom-up for modeling the domain ontology and the argumentation rules. Its sample consists in 27 decisions of Italian case-law, from different courts (Tribunal, Court of Appeal, and Cassation Court) but all concerning the same legal subject: consumer law.2 The research relies on the previous efforts of the community in the field of legal knowledge representation (Hoekstra et al. 2009) and rule interchange for applications in the legal domain (Gordon et al. 2009). The issue of implementing logics to represent judicial interpretation has already been faced, albeit only for the purposes of a sample case. The aim of the present research is to apply these theories to a set of real legal documents, stressing the definitions of OWL axioms as much as possible in order to provide a semantically powerful representation of the legal document for an argumentation system that relies on a defeasible subset of predicate logics.
The Legal Ontology (Palmirani and Ceci 2012) creates an environment where the knowledge extracted from the decision’s text can be processed and managed in order to perform deeper reasoning on the interpretation instances grounding the decision itself. This reasoning is based on the argumentation model of the Carneades Argumentation System.3 The framework is capable of creating argumentation graphs in favour (pro) or against (con) a given legal statement, not only when all the premises for the argument are accepted in the knowledge base: Carneades is in fact capable of suggesting incomplete arguments (Ceci and Gordon 2012), thus highlighting critical aspects of the case which were not been taken into consideration by the judge (in the precedent case) or by the user (in the query). This means that, given a set of judicial decision encoded in the OWL ontology, the program is capable not only to represent the argumentation path followed by the judge, but also possible alternative paths that lead to different outcomes.
32.2 Representing Argumentation Patterns
32.2.1 Introduction
Any standard devoted to the representation of legal rules should include a unification of the logic layer into a single language. Some argue that the various aspects of argumentation can be properly represented through logics: in particular, Governatori (2011) shows how proof standards proposed in the Carneades framework correspond to some variants of defeasible logics, which could imply that an implementation of defeasible logics is able to compute acceptability of arguments. However this doesn’t seem to be the case, in the light of the following considerations:
Logics provide abstract formulas to represent relationships between concepts. With fine tools such as defeasible logics it is possible to successfully represent the complex relations between legal rules, but can they manage the application of these rules, a fundamental step towards the computation of the acceptability of an argument? In theory it could be the case, since the act of substitution of abstract symbols in formulas with the values of the situation we want to compute should be an automatic process where it doesn’t matter which material concept is added as the interpretation of that abstract symbol. For example, if we have a + b = c, we can interpret this simple rule as meaning many different things (for example a = 1, b = 2, c = 3, + = addition, or a = blue, b = yellow, c = green and + = mix) and this would not affect the truth function of the equation. On the contrary, in the concrete legal field the single elements bring with them particular conditions (minor rules or meta-rules), assumptions, exceptions, values, which can significantly alter the outcome of the abstract formula representing the rules.
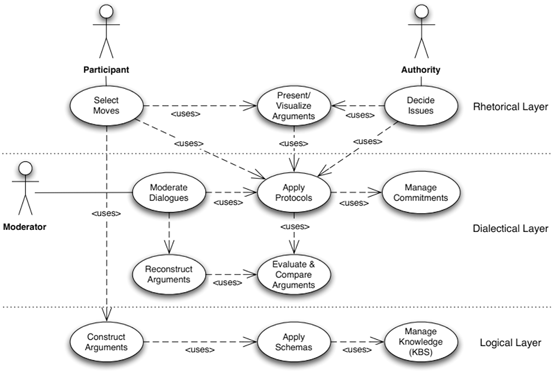
Most of the application scenarios of legal language are centered on dialogues with two or more parties in which claims are made and competing arguments are put forward to support or attack these claims (this includes judgements, which are the focus of this paper, but also parliamentary debates and other legal acts). Following Walton, we recognize there are several kinds of dialogues, with different purposes and different protocols (Fig. 32.2). This view of arguments as dialogues (or processes) contrasts with the mainstream, relational conception of argument in the field of computational models of arguments, typified by Dung (1995), where argumentation is viewed not as a dialogical process for making justified decisions which resolve disputed claims, but as a method for inferring consequences from an inconsistent set of propositions. To see the difference between these conceptions of arguments, notice that a proposition that has not been attacked is acceptable in this relational model of argument, whereas in most dialogues a proposition that has not been supported by some argument is typically not acceptable, since most protocols place the burden of proof on the party that made the claim.
Before exploring those two themes, a preliminary presentation of argument schemes is necessary.
32.2.2 Argumentation Schemes and Critical Questions
An argumentation scheme is a pattern of reasoning used in everyday conversation and other contexts, such as legal and scientific argumentation. Argumentation schemes serve the same purpose as their ancestors, the τόποι (topics) of Aristotle: they are useful to create, evaluate and classify arguments. In recent times, the Artificial Intelligence field has become increasingly interested in argumentation schemes, due to their potential for making improvements in the reasoning capabilities of agents (Verheij 2003; Garssen 2001; Dung and Sartor 2011). Two functions of argumentation schemes can be distinguished in the legal field: as argument patterns useful for reconstructing arguments from natural language texts, and as methods for generating arguments from argument sources, such as legislation or precedent cases.
In argumentation theory, argumentation schemes are evaluated through a set of critical questions4 (CQs), specific for each scheme. Each question reveals possible weak points in the argumentation, and if not answered adequately may render that specific argument useless in supporting the speaker’s position in the dialogue. Evidently, critical points in arguments should be formalized in a dialogical structure, in order to maintain the notion of defeasibility of every argument in the scheme, including those introduced to answer one or more critical questions. The example from Walton’s analysis of expert opinion (Walton 1997) perfectly explains this dialogical structure. Following is the model of argument from expert opinion:
Source E is an expert in domain D
E asserts that proposition A is known to be true (or false)
A is within D
Therefore, A may plausibly be taken to be true (or false).
As shown by experiments in social psychology, however, there is a tendency to defer to experts, sometimes without questioning, resulting in fallacious appeals to authority. Many circumstances could prevent the apparently deductive conclusion that “if E says A, then A is true”: in particular, epistemic closure in an expert field is far from truth, and therefore an expert can never be considered as knowing everything in a domain, and neither can its opinion be deductively true beyond challenge. Thus for many (if not all) appeals to the expert opinion, the deductivist approach does not work. Critical questions are used to ease tensions between forms of argument that are clearly reasonable in some instances, but that cannot be analysed as deductively valid (Reed and Walton 2001). Walton (1997) identifies six basic critical questions matching the appeal to expert opinion:
a.
How credible is E as an expert source?
b.
Is E an expert in D?
c.
Does E’s testimony imply A?
d.
Is E reliable?
e.
Is A consistent with the testimony of other experts?
f.
Is A supported by evidence?
Please notice that, in many cases, asking one of the basic critical questions above will lead to critical sub-questions at a deeper level of examination. This is one way to create argumentation graphs (Gordon 2010).